A "functional" Transformation

One of the biggest topics in a secondary mathematics class is function transformations (horizontal/vertical shift, compressions, stretches, etc.) Usually students will memorize the rules rather than understand the reasoning. I needed a way to enhance the lesson to allow students room to investigate these concepts and draw their own connections and conclusions - time to spice things up with technology!

Topic: Intro to Transformations

Essential Question: How can I transform ANY function?

Materials:

• Desmos Activity - Make a copy if you'd like to make some adjustments! (link)
• Student Notes - Google Slides (link) Click [Use Template] to make your own copy in your Google Drive.
• 1 device per 2 students - I recommend a Chromebook/computer.
• Recommendation: a Google Classroom to share the notes with each student.

Student Notes (Google Slides) Preview

Teacher Notes:

1. Assign the notes in Google Classroom where each student gets a copy.
2. Go to teacher.desmos.com. If you don't have one, create an account! I recommend with a Google Account to make the sign-in easier.
3. Open the Desmos Activity (Intro to Transformations) above. 
4. Click on the teal [Create Class Code]. This will need to be copied on Slide 1 in the Google Slides. This is how students will access the activity.

   

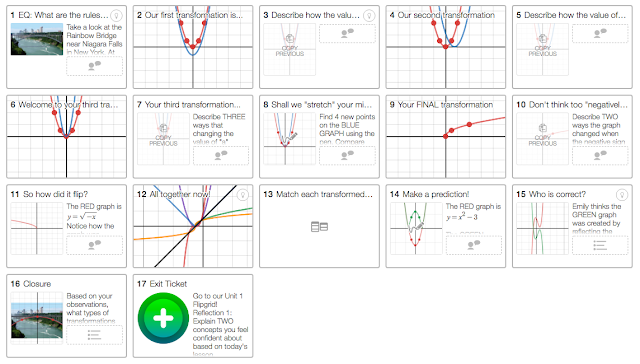   Desmos Activity (Preview)

5. Students will partner up and bring their computer/Chromebook. Each student can login so that everyone answers the activity questions and can take screenshots to add to their Google Slides for their notes.
6. Encourage students to take their time exploring each transformation carefully. They may struggle understanding shifting left and right. I related it back to the distance formula - (x - 3) moves RIGHT 3, not LEFT. 
   
7. The Exit Ticket/Reflection piece is on Flipgrid. Create a TOPIC in Flipgrid where students can respond.
8. TIP: I created a topic for EACH UNIT in my class so that students could see how their questions and reasoning improved as the unit continued. 

My Reflection:

• Since the remaining of the year (and future mathematics courses) depended on this lesson, I felt strongly about student exploring it on their own. I tried teaching the rules one year and it only set them up for failure later. 
• I'm currently reading Shake Up Learning by the infamous Kasey Bell of Texas. In her first couple of chapters, she stresses the importance of integrating 21st century skills into as many lessons as possible - hence, shake things up! Students will be asked to analyze critically and shoving rules in their faces wasn't accomplishing this and was NOT preparing my students for their future careers. Careers where thinking on their own and creating their own connections would be a requirement.
• One of my biggest fears with "shaking up their learning" is that my students won't learn the concepts "correctly". What if they misunderstand and I don't catch it?! In my mind, technology allows me to assess formatively more often, therefore I can check understanding frequently. When my concern of "learning incorrectly" snuck in to my mind, I forced myself to remember Jo Boaler - making mistakes is a learning opportunity. Even more so, making mistakes will make their brains grow.